Signal Distortion in Optical Fibers - Attenuation | NOTES | ECETOTAL

Table of Contents

• Signal Distortion
• Condition for signal distortion-less transmission
• Special nature of Optical Signal
• Attenuation on Optical Fiber
• Material Loss
• Scattering Loss
• Micro-Bending Losses
• Radiation or Bending Loss

Signal Distortion

A signal is said to be undistorted if it is delayed in time and a scaled version of the original signal. Let a system has an input signal and output signal. Then for distortion-less system

y(t) = kx (t-Ꞇ)...............(1)

Where k is a scaling constant and Ꞇ is the time delay.

Condition for signal distortion-less transmission

To see the condition in the frequency domain let us take the Fourier transform of Eqn (1). If Y(ω) is the Fourier transform of y(t) and X(ω)is the Fourier transform of x(t), we get, 

Y(ω) = ke^-jωꞆX(ω)................(2)

That means if a system has a frequency response ke^-jωꞆ then it does not produce any distortion of the signal. In other words for a system to be distortion-less, its frequency response must satisfy two conditions.

1. The amplitude response must be constant (k should be independent of frequency).
2. The phase response should be linear (Ꞇ should be constant).

Why does the signal gets distorted while propagating on optical fiber?

1. The loss of optical fiber is wavelength-dependent. That makes k a function of frequency. This is called the attenuation of the fiber.
2. Different wavelengths and different modes of the optical fiber travel with different speeds and therefore have a delay that is wavelength-dependent. This phenomenon is called dispersion. 

• So, in general, the signals get distorted on an optical fiber because different frequency components undergo different attenuation and different delays.
• For an optical communication link, the highest data rate is about 10GHz. This translates approximately to a wavelength range of 0.1nm. For all practical purposes, the loss of the optical fiber can be assumed constant over a bandwidth of 0.1nm. Therefore, we note that the uniform amplitude response condition is well satisfied for the optical fiber. The signal depending upon the loss may reduce in amplitude but there is no distortion of the signal due to the loss. 
• The typical optical wavelength is about 1550nm and the signal bandwidth is about 0.1nm. That means the optical system is a very narrow band system, the fractional bandwidth is << 1. One would then wonder whether over such a narrow bandwidth the linearity of the phase response is violated! Or would the velocity differ significantly over such a narrow bandwidth to give substantial distortion! The answer to this would be ‘NO'. However, before we conclude this let us look at the special nature of the optical signals.

Special nature of Optical Signal

The Figure gives the spectra of modulating signal and the carrier for two types of communication, radio communication, and optical communication.

Special nature of the optical signal

There is a marked difference between the two cases which can be summarized as follows:

1. For radio communication, the intrinsic spectral width of the carrier is very small compared to the spectral width of the modulating signal. Therefore the bandwidth of the modulated signal is almost equal to the BW of the modulating signal. (twice if the signal is simple AM). Also, the shape of the spectrum of the modulated signal is almost the same as that of the modulating signal. So in radio communication, the spectrum of the modulating signal is preserved, except that it is shifted by the carrier frequency.
2. For optical communication, the scenario is quite opposite. A typical optical source like LED has an intrinsic spectral width of 30-60nm (approximately 4000 to 8000GHz), and a source like a laser diode has a spectral width of 2-3nm (approximately 250 to 400 GHz). That means for optical communication the spectral width of the carrier is much greater than the spectral width of the data. The bandwidth of the modulated signal then is almost equal to the bandwidth of the carrier with hardly any signs of the data. So, in optical communication, the spectrum cannot be used to recover the data. The demodulation, therefore, has to be done only in the time domain. This is possible only by AM scheme where the signal can be recovered by a primitive technique of envelop detection.

• The optical communication system hence cannot be treated online similar to that used for the normal radio communication link. Also since the modulated signal has a spectral width of few nm, the fractional bandwidth is not as small as it appeared earlier.
• The optical communication system can be looked at as a parallel multiple channel transmission of carriers spreading over the bandwidth of the carrier. One can then say that the distortion of the signal in optical communication is due to the differential delay of the signal riding over different carriers within the spectral width of the carrier.
• The signal pulse then goes on spreading as it travels along with the optical fiber. The pulse broadening is proportional to the distance and it is also proportional to the spectral width of the carrier. This phenomenon is dispersion.
• So we conclude that when a signal pulse travels on an optical fiber it goes on broadening due to dispersion and goes on reducing in amplitude due to attenuation, as shown in Fig. After a certain distance the pulse shape, is completely distorted not to resemble the original pulse shape.

Attenuation on Optical Fiber

The signal on optical attenuates due to the following mechanisms :

1. Intrinsic loss in the fiber material.
2. Scattering due to micro irregularities inside the fiber.
3. Micro-bending losses due to micro-deformation of the fiber.
4. Bending or radiation losses on the fiber.

Attenuation on Optical Fiber

The first two losses are intrinsically present in any fiber and the last two depend on the environment in which the fiber is laid.

Material Loss

1. Due to impurities: The material loss is due to the impurities present in glass used for making fibers. In spite of best purification efforts, there are always impurities like Fe, Ni, Co, Al which are present in the fiber material. Fig. shows attenuation due to various molecules inside the glass as a function of wavelength. It can be noted from the figure that the material loss due to impurities reduces substantially beyond about 1200nm wavelength.
2. Due to OH molecule: In addition, the OH molecule diffuses in the material and causes absorption of light. The OH molecule has the main absorption peak somewhere in the deep infra-red wavelength region. However, it shows a substantial loss in the range of 1000 to 2000nm.
3. Due to infra-red absorption: Glass intrinsically is a good infra-red absorber. As we increase the wavelength the infra-red loss increases rapidly.

Scattering Loss

• The scattering loss is due to the non-uniformity of the refractive index inside the core of the fiber. The refractive index of an optical fiber has a fluctuation of the order of 10^-4 over spatial scales much smaller than the optical wavelength. These fluctuations act as scattering centers for the light passing through the fiber. The process is, Rayleigh Scattering. A very tiny fraction of light gets scattered and therefore contributes to the loss.
• The Rayleigh scattering is a very strong function of the wavelength. The scattering loss varies as (Lamda) Λ^-4. This loss therefore rapidly reduces as the wavelength increases. For each doubling of the wavelength, the scattering loss reduces by a factor of 16. It is then clear that the scattering loss at 1550nm is about a factor of 16 lower than that at 800nm. The following Fig. shows the infrared, scattering, and total loss as a function of wavelength. 

scattering and the total loss as a function of wavelength

• It is interesting to see that in the presence of various losses, there is a natural window in the optical spectrum where the loss is as low as 0.2-0.3dB/Km. This window is from 1200nm to 1600nm. 
• There is a local attenuation peak around 1400nm which is due to OH absorption. The low-loss window, therefore, is divided into sub-windows, one around 1300nm and the other around 1550nm. In fact, these are the windows that are the II and III generation windows of optical communication.

Micro-Bending Losses

• While commissioning the optical fiber is subjected to micro-bending as shown in Fig.

Micro bending losses

• The analysis of micro-bends is a rather complex task. However, just for basic understanding of how the loss takes place due to micro-bending, we use following arguments.
• In a fiber, without micro-beads, the light is guided by total internal reflection (ITR) at the core-cladding boundary. The rays which are guided inside the fiber have an incident angle greater than the critical angle at the core-cladding interface. In the presence of micro-bends, however, the direction of the local normal to the core-cladding interface deviates and therefore the rays may not have an angle of incidence greater than the critical angle and consequently will be leaked out.
• A part of the propagating optical energy, therefore, leaks out due to micro-bends.
• Depending upon the roughness of the surface through which the fiber passes, the micro-bending loss varies.
• Typically the micro-bends increase the fiber loss by 0.1-0.2 dB/Km. 

Radiation or Bending Loss

• While laying the fiber the fiber may undergo a slow bend. In micro-bend, the bending is on micron-scale, whereas in a slow bend the bending is on cm scale. A typical example of a slow bend in the formation of an optical fiber loop.
• The loss mechanism due to bending loss can be well understood using the modal propagation model.
• As we have seen, the light inside a fiber propagates in the form of modes. The modal fields decay inside the cladding away from the core-cladding interface. Theoretically, the field in the cladding is finite no matter how far away we are from the core-cladding interface. Now, look at the amplitude and phase distribution for the fibers which are straight and which are bent over a circular arc as shown in Fig.

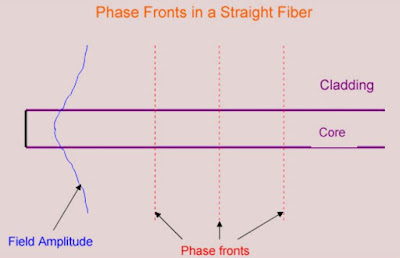Phase Fronts in Straight Fiber

• It can be noted that for the straight the phase fronts are parallel and each point on the phase front travels with the same phase velocity.

Phase Fronts for Bent Fiber

• However, as soon the fiber is bent (no matter how gently) the phase fronts are no more parallel. The phase fronts move like a fan pivoted to the center of curvature of the bent fiber (see Fig.). Every point on the phase front consequently does not move with the same velocity. The velocity increases as we move radially outwards the velocity of the phase front increases. Very quickly we reach a distance xc from the fiber where the velocity tries to become greater than the velocity of light in the cladding medium.
• Since the velocity of energy can not be greater than the velocity of light, the energy associated with the modal field beyond xc gets detached from the mode and radiates away. This is called bending or radiation loss.

Following important things can be noted about the bending loss :

1. The radiation loss is present in every bent fiber no matter how gentle the bend is.
2. Radiation loss depends upon how much is energy beyond.
3. For a given modal field distribution if reduces, the radiation loss increases. The reduces as the radius of curvature of the bent fiber reduces, that is the fiber is sharply bent.
4. For a given that is for a given fiber bent, if the field spreads more in cladding, the bend loss increases. We know from the modal field analysis that the lower-order modes are more confined to the core, that is their fields decay rapidly in the cladding, and the higher-order modes have more slowly decaying energy in the cladding. The higher-order modes hence are more susceptible to radiation loss compared to the lower-order modes.
5. The number of modes, therefore, reduces in a multimode fiber in presence of bends. 

ALSO READ What is a DIODE?

ALSO READ What is SEMICONDUCTOR (SC)?

ALSO READ Definition of Electronic circuits